
Water Flow into Deep Creek Lake
The chart shown in Figure 1 has been seen in several DNR briefings and reports (I don’t have any references). It is noted that it depicts rainfall on the lake surface for 1970 to 2011 for the months of July thru September.
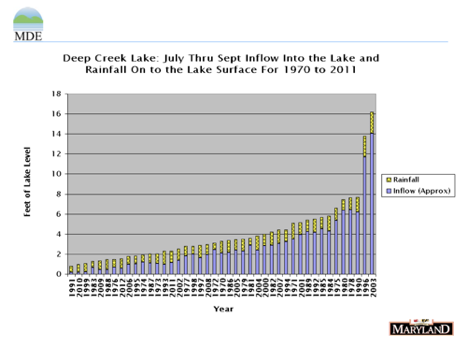
Figure 1 - Annual Water Flow into Deep Creek Lake
As far as I know, there were no raingages on the lake. I don’t know how the numbers were obtained nor the rationale for the “Inflow (Approx)” variable but it does not pass a sanity check because of the following reasons.
I would have expected that the ratio of Rainfall to Inflow to be roughly the same. After-all, the only water coming into the lake is from streams, groundwater and runoff. Streams originate in the watershed and are all supplied by ground water in their respective watersheds, and these in turn by the rainfall.
When computing the annual amount of water consumed by the hydroelectric facility one gets roughly 20 ft (see Appendix A). Since the MWhrs generated is pretty much the same every year (From a Stone & Webster report which states that this is a historical average), the amount of water that must feed into the lake must be the same on a yearly basis also, and must be approximately 20 ft.
Perhaps the inflow is from streams only? The USGS flow gages have been around for a long time.
Elsewhere on this website is a figure that shows the daily incremental rainfall for the years 1994-2016., and other analyses involving rain.
Appendix A
How much generating capacity is one ft of water for Brookfield?
1. Volume of 1 ft of lake water: 3,900 acres (lake surface area) x 43,560 ft2/acre x 1 ft = 1.7x108 ft3
2. The capabilities of the turbines: 600 ft3/sec produces 18 MW (specifications for the turbines; I found somewhere a suggestion that they may be operating at 310 cfs per turbine rather than the nominal design value of 300 cfs)
3. The number of hours the turbines operate to consume one foot of water: 1 ft implies ⇒ 1.7x108 ft3/600 ft3/sec x 1hr/3,600 sec ⇒ 78.7 hrs of operation
4. The total amount of energy produced by one foot of water: 78.7 hrs x 18 Mw ⇒ 1,416 MWhrs
5. The annual production of energy by the facility: Average generation per year is 28,500 MWhrs(from a Stone & Webster report commissioned by Reliant, the predecessor to Brookfield)⇒ 28,500 / 1,416 = 20 ft of water.
6. Average rainfall per year The average amount of rainfall per year is 48 inches. The total area of the watershed has a drainage area of about 41,410 acres. Hence the total amount of water that could wind up in the lake is (48/12)x(41,410+3,900) = 181, 200 acre-feet of water.
For a lake surface area of 3,900 acres, if all the water would enter the lake, the amount of water depth removed from the lake must be: 181,200/3,900 = 46.5 ft!?
Note that Figure 1 is quite different.
PLV
First Published: 1/3/2013